This page contains the GCSE AQA Mathematics Vectors Questions and their answers for revision and understanding Vectors.
A vector is something with both magnitude (size) and direction. On a diagram, they are denoted by an arrow, where the length of the arrow tells us the magnitude and the way the arrow is pointing tells us the direction.
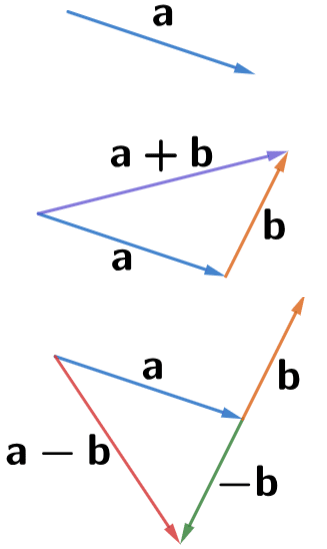
When we add vectors, we add them end-to-end. For example, if you add two vectors a and b, then the result is the vector a+b, which takes you from the start of a to the end of b (right).
The negative of a vector has the same magnitude of the original vector, it just goes in the exact opposite direction. When we subtract vectors, for example a−b, we add on the negative of the vector that is being subtracted, i.e. we add the vector −b onto the vector a, as seen in the picture.
We can multiply a vector by a number. As with normal multiplication, it’s just the same as adding a number to itself multiple times, so the result of multiply a by 3 is
3a=a+a+a
Then, they are added end-to-end, just how we’ve already seen. Note: all vectors here are written in bold. When you’re writing this by hand, you should underline each letter that represents a vector.
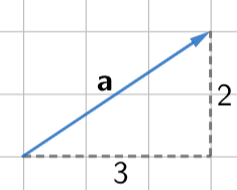
Vectors are often split up into two parts – an x part, which tells us how far the vector moves left or right, and a y part, which tells us how far a vector moves up or down. When splitting up vectors like this, we express them as column vectors, where the top number is the x part and the bottom number is the y part.
For example, the vector a goes 3 spaces to the right and 2 spaces up, so would be expressed like (3/2). If the vector goes left, the x value is negative, and if it goes down, the y value is negative.
To add/subtract column vectors, we add/subtract the x and y values separately. For example,
(−3/4)+(5/2)=(2/6)
To multiply a column vector by a number, we multiply both values in the vector by that number, e.g.
5×(2/−3)=(10/−15)
It’s important to understand that the vectors you see diagrams of and vectors written in column form are just different ways of working with the same thing. Suppose you have two column vectors, which you then add together to get another vector. If you then drew those two column vectors on a diagram and added them end-to-end (like we saw above), the resulting vector would be precisely what you got when you added the two column vectors.
For the foundation course it is sufficient to have a good understanding how to represent vectors on a diagram and as a column, as well as knowing how to add/subtract/multiply them in both forms. However, for the higher course, there is a little more.
Firstly, a vector that goes from some point A to another point B may be denoted like AB. If a vector starts from the origin and goes to some point, say A, it will be written with an O, like OA.
The way to add these vectors is explained by the picture on the right. As you can see, we still add them end-to-end. So, if you add the vector AB to the vector BC, then the result is a vector that starts at A and ends at C, thus is denoted by AC. The fact that it goes via B doesn’t matter, what matters is where it starts and ends.
The easiest way to see a typical structure of a higher vector question is to see an example. Let’s go.
Example: In the diagram below, we have vectors 
and 
are shown. Point DD lies on the line BC such that BD:DC=1:3. Write the vector 
in terms of a and b.
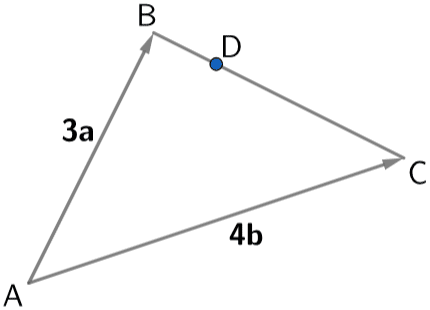
There’s a lot going on here, but we’ll break it down. To find , we are going to add the vectors
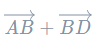
Added end-to-end, they will give us a vector that starts at A and ends at B. For the first one, we don’t need to do anything – we know AB=3a. The second part will require some work.
Firstly, recognise that if BD:DC=1:3, then D is 14 of the way along the line from B to C. Therefore, if we get the vector that goes from B to C and divide it by 4, we have BD. Now, to get from B to C using the vectors we’ve been given, we can go via A. To get from B to A, we have to go backwards along , so we will take the negative of it. Therefore, we get
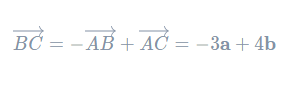
Dividing this by 4, we get
Therefore, adding this toAB, we get the answer to be
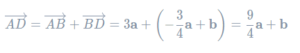
Thus, we have our answer in terms of a and b.
Note: another common type of question is “prove one vector is parallel to another”. To do this, you work out both vectors in questions, and then if one is multiple of the other, they are parallel.